Answer:
The length of the bold arc is approximately 13.4 mi
Explanation:
The radius of the circle having the arc, r = 17 mi
Therefore, the circumference of the circle, 'C', is given as follows;
C = 2·π·r
∴ C = 2×π×17 = 34·π
The angle subtended by the arc = 45°
The sum of the angles at the center of the circle = 360°
By similarity, the ratio of the length of the bold arc to the circumference of the circle = The ratio of the angle subtended by the arc to the sum of the angles at the center of the circle
Mathematically, we have;
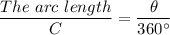
Therefore, we get;
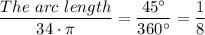
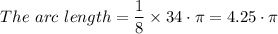
The length of the bold arc = 4.25·π mi ≈ 13.4 mi (by rounding off the answer to the nearest tenth).