Question

Linda opened a savings account and deposited 100.00 as principal the account earns 11%interest compounded monthly what is the balance after 9 years

Answer 1

The balance on the account after 9 years is 268.00

Here, we want to use the compound interest formula to know the yield on the savings accoint after a period of nine years

We use the compound interest formula as follows;

`A\text{ = P( 1 + }(r)/(n))^(nt)`

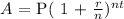
where A is the balance on the account after the period of 9 years

P is the principal which is the amount deposited which is 100.00

r is the interest rate which is 11% which is same as 11/100 = 0.11

n is the number of times per year that the interest is compounded. In this case, since it is monthly, then the interest is compounded 12 times a year

t is the number of years which is 9

Thus, inputing these values in the compound interest equation, we have;

`\begin{gathered} A\text{ = 100 (1 + }(0.11)/(12))^(12*9) \\ \\ A=100(1+0.0091667)^(108) \\ \\ A=100(1.0091667)^(108) \\ \\ A\text{ = 267.913} \\ \\ To\text{ the nearest whole unit, it is 268.00} \end{gathered}`