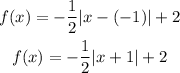Answer:
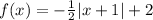
Step-by-step explanation:
Given the equation of the function graphed in blue, which is also the parent function;
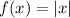
An equation of an absolute function after a transformation will be in the form;
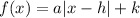
when a > 0, the graph will open upwards
a < 0, the graph will open downwards
0 < a < 1, the graph will be compressed
a > 1, the graph will be stretched
h > 0, the graph will be translated h units to the right
h < 0, the graph will be translated h units to the left
k > 0, the graph will be translated k units upwards
k < 0, the graph will be translated k units downwards
Looking at the function graphed in red, we can notice the below;
*The graph opens downwards, this shows that a is negative.
*The graph moves 2 units upwards, this shows that k = 2
*The graph moves 1 unit to the left, this shows that h = -1
*The graph is compressed by a factor of 1/2, therefore a = 1/2
If we substitute these values into the equation above, we'll have;