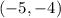Question

Consider the equation belowY=3x²+30x+71Use completing the square to rewrite the given equation and reveal the extreme valueY=3(x+___)²+____The extreme value of the equation is at (___ , ___)

Answer 1

Given:

`y=3x^2+30x+71`

To find:

Rewrite the equation using the completing the square method.

Step-by-step explanation:

It can be written as,

`\begin{gathered} y=3(x^2+10x)+71 \\ =3(x^2+10x+5^2-5^2)+71 \\ y=3[(x^2+10x+5^2)-25]+71 \end{gathered}`

Using the algebraic identity,

`a^2+2ab+b^2=(a+b)^2`

We can write,

`\begin{gathered} y=3[(x+5)^2-25]+71 \\ y=3(x+5)^2-75+71 \\ y=3(x+5)^2-4 \end{gathered}`

Therefore, the equation becomes,

`y=3\left(x+5\right)^(2)-4`

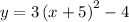
It is of the form,

`y=a\left(x-h\right)^2+k,(h,k)\text{ is the extreme value of the function}`

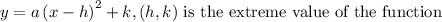
So, the extreme value of the equation is at

`(-5,-4)`

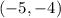
Final answer:

The equation is written as,

`y=3\left(x+5\right)^(2)-4`

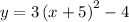
The extreme value of the equation is at

`(-5,-4)`