Answer:
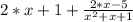
Explanation:
Where, the quotient is 2·x + 1, and the reminder is 2·x - 5
Hence, the above is correct is as follows:
The given dividend is 2·x³ + 3·x² + 5·x - 4
The divisor is x² + x + 1
By long division of a polynomial we have;
2·x + 1 [Quotient]
(2·x³ + 3·x² + 5·x - 4) ÷ (x² + x + 1 )
2·x³ + 2·x² + 2·x
0 + x² + 3·x - 4
x² + x + 1
0 + 2·x - 5
Thurs, we have:

Hence, the correct answer is
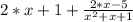
[RevyBreeze]