Question

I don't understand the as the visualas an equationsolution to platform

Answer 1

This is the visual

The length of the rectangle is 3 more than twice the width

Let the width of the rectangle = x

The length = 2(x) + 3 = 2x + 3

Its area = length x width

Area = (2x + 3) x x

Area = x(2x + 3)

The area of the rectangle = 44 square meters

Equate the area by 44

x(2x + 3) = 44

The equation is x(2x + 3) = 44

Let us solve the equation

Multiply the bracket by x

`\begin{gathered} x(2x)+x(3)\text{=44} \\ 2x^2+3x=44 \end{gathered}`

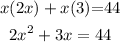
Subtract 44 from both sides

`\begin{gathered} 2x^2+3x-44=44-44 \\ 2x^2+3x-44=0 \end{gathered}`

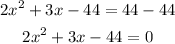
Factorize it into two factors

`\begin{gathered} 2x* x=2x^2 \\ -4*11=-44 \\ 2x*-4=-8x \\ x*11=11x \\ 11x-8x=3x \end{gathered}`

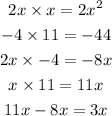
The factors are (2x + 11)(x - 4)

(2x + 11)(x - 4) = 0

Equate each factor by 0 to find x

x - 4 = 0

Add 4 to both sides

x - 4 + 4 = 0 + 4

x = 4

The width = 4

The length = 2(4) + 3 = 8 + 3 = 11

The dimensions are 4 m and 11 m

The second factor will give a negative value of x, then we will refuse it, no dimension with a negative value