Question

Find the MAD of this set of data.6, 12, 10, 9, 8, 6, 2, 4, 8, 153.582.89

Answer 1

Mean absolute deviation (MAD) of a data set is the average distance between each data value and the mean.

We must first find the mean of the data set:

`\begin{gathered} \bar{x}\text{ = }(6+12+10+9+8+6+2+4+8+15)/(10) \\ =\text{ 8} \end{gathered}`

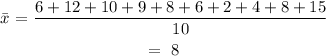
D1 = 6-8 = -2 = 2

D2 = 12-8 = 4

D3 = 10-8 = 2

D4 = 9-8 = 1

D5 = 8-8 = 0

D6 = 6-8 = -2 = 2

D7 = 2-8 = -6 =6

D8 = 4-8 = -4 = 4

D9 = 8-8 = 0

D10 = 15-8 = 7

`\begin{gathered} MAD\text{ = }(2+4+2+1+0+2+6+4+0+7)/(10) \\ =2.8 \end{gathered}`

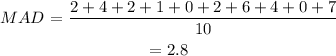
Correct option: MAD = 2.8