Answer:
y=1/3x+1
Step-by-step explanation:
Two lines are parallel if they have the same slope.
Comparing the equation of the line with the slope-intercept form:
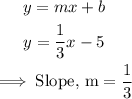
Therefore, the line parallel to it has a slope of 1/3.
Thus, using the slope-point form, we find the equation of a line with a slope of 1/3 and passing through (3,2).

The equation of the parallel line is y=1/3x+1.