Question

Part 1 :What conjecture can you make about the sum of the first 41 positive odd numbers? make a conjecture. choose the correct answer below.1. the sum is the product of the number of terms and two.2. the sum is equal to the number of terms squared.3. the sum is the product of the number of terms and the number of terms plus one.4. the sum is the product of the number of terms and the number of terms minus one.-Part 2 : what is the sum in a whole number?

Answer 1

Step 1:

In this question, we are meant to make a conjecture about the sum of the first 41 positive odd numbers:

`1,\text{ 3, 5 , 7, 9, 11, }\ldots.`
`\begin{gathered} \text{Using the sum of the first 41 terms ( Arithmetic progression)} \\ S_{\text{n }}=\text{ }(n)/(2)\lbrack\text{ 2a + ( n - 1) d\rbrack} \\ Sn=\text{ Sum of n terms} \\ n\text{ = number of terms = 41} \\ \text{a = first term = 1} \\ d\text{ = common diference = 3 - 1 = 2} \end{gathered}`

Step 2:

`\begin{gathered} S_(41)=\text{ }(41)/(2)\lbrack\text{ 2(1) + ( 41- 1) 2\rbrack} \\ S_(41)=\text{ }(41)/(2)\lbrack\text{ 2 + ( 40 x 2 ) \rbrack} \\ S_(41)=\text{ }(41)/(2)\lbrack\text{ 2 + 80\rbrack} \\ S_(41)=\text{ }(41)/(2)\text{ X }(82)/(1) \\ S_(41)\text{ = 41 X 41 = 1681} \end{gathered}`

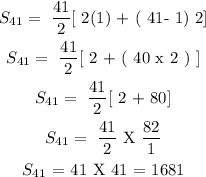
Step 3:

Part 1: From the above calculations, we can see clearly that:

This corresponds to OPTION 2 :

The sum is equal to the number of terms squared.

Part 2: The sum in a whole number is 1681