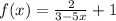Given: The derivative of the function is given as

The antiderivative is guessed as
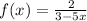
Required:
(a) Is the guess correct?
(b) Is it the only possible answer?
(c)Find another anti derivative if possible.
Step-by-step explanation:
To find the antiderivative, we must integrate the derivative
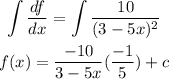
where c is a constant.
Now,

(a)
For c = 0
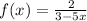
So the guess is correct.
(b)
There can be many other answers, by putting different values of c, we can get different antiderivatives.
So, student answer is not the only possible answer.
(c)
For any other anti-derivative, put c = 1
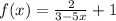
Final Answer:
(a) Yes
(b) No
(c)