Question

The area of a rectangle is 4320 units. The ratio of the width to the length is 5:6. Find the length and width.

Answer 1

The general expression for the area of rectangle is : Length x Width

The area of a rectangle is 4320 units.,

The ratio of the width to the length is 5:6.

Let the ratio constant = x

So, Length =6x and Width = 5x

Area of rectangle of the given dimension :

`\begin{gathered} \text{ Area of rectangle = Length x Width} \\ \text{ Area of rectangle = 6x}*5x \\ \text{ Area of rectangle = }30x^2 \end{gathered}`

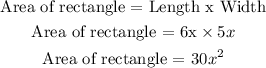
as it is given that the area is 4320

`\begin{gathered} 30x^2=4320 \\ \text{Simplify for x:} \\ x^2=(4320)/(30) \\ x^2=144 \\ x=\sqrt[]{144} \\ x=\pm12 \\ \text{But as measurement cannot be negative so} \\ x=12 \end{gathered}`

Length = 6x

Substitute the value of x = 12

Length =6(12)

Length =72

Width = 5x

Substitute the value of x = 12

Width = 5x

Width =5(12)

Width = 60

Answer : length = 72, width = 60