Answer:
![((f)/(g))(x)\text{ = }\frac{\sqrt[3]{3x}}{3x+2},\text{ x}\\e\text{ -}(2)/(3)](https://img.qammunity.org/2023/formulas/mathematics/college/rt6jj8fsswxyfevnm5x8n2jy7cgq42ubwp.png)
Step-by-step explanation:
Here, we want to evaluate the function division
Mathematically, we have this as follows:
![((f)/(g))(x)\text{ = }(f(x))/(g(x))\text{ = }\frac{\sqrt[3]{3x}}{3x+2}](https://img.qammunity.org/2023/formulas/mathematics/college/njnw49elv4xzm4ihnibho47la9v8m1pg1s.png)
Finally, we need to get the domain restriction
To get the domain restriction, we need to find the value of x under which the denominator becomes zero
Mathematically, we have this as:
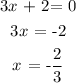
Thus, we have the correct representation as:
![((f)/(g))(x)\text{ = }\frac{\sqrt[3]{3x}}{3x+2},\text{ x}\\e\text{ -}(2)/(3)](https://img.qammunity.org/2023/formulas/mathematics/college/rt6jj8fsswxyfevnm5x8n2jy7cgq42ubwp.png)