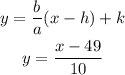Answer:
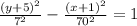
![\text{Foci = (}-1,\text{ -7}\sqrt[]{101}-5)\text{ and (}-1,\text{ -5+7}\sqrt[]{101})](https://img.qammunity.org/2023/formulas/mathematics/college/4rli5iin1dyc54tnsdvik6m2mdjmtbyc0u.png)
The vertex = (-1, -12) and (-1, 2)
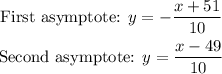
Step-by-step explanation:
The given equation of the hyperbola is:
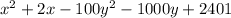
The standard form of the equation of a hyperbola is of the form

where (h, k) is the center of the hyperbola
The vertex = (h, k-b) and (h, k+b)
The given equation can be re-written as:
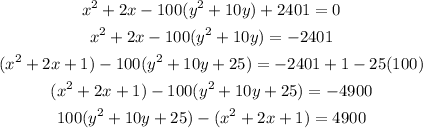
This can be further simplified into:
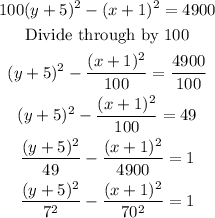
Therefore, the standard form of the given parabola equation is:
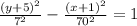
The center (h, k) = (-1, -5)
(a, b) = (70, 7)
The vertex = (-1, -5-7) and (-1, -5+7)
The vertex = (-1, -12) and (-1, 2)
The foci = (h, k-c) and (h, k+c)
![\begin{gathered} c=\sqrt[]{a^2+b^2} \\ c=\sqrt[]{70^2+7^2} \\ c=7\sqrt[]{101} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/30ts6w2sxm802ld5u39xtgrr8wp1gdodqr.png)
![\text{Foci = (}-1,\text{ -7}\sqrt[]{101}-5)\text{ and (}-1,\text{ -5+7}\sqrt[]{101})](https://img.qammunity.org/2023/formulas/mathematics/college/4rli5iin1dyc54tnsdvik6m2mdjmtbyc0u.png)
The first asymptote:

Second asymptote