Question

Hi, can you help me answer this question, please, thank you:)

Answer 1

Given:

1% rate of defects

There are a total of 21 tablets tested

We use binomial probability distribution to solve this particular problem.

The general formula for the binomial distribution is:

`P\mleft(x\mright)=^nCx.p^x\cdot\mleft(1-p\mright)^(n-x)`

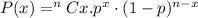
where x is the number of successes in n trials (In this problem, x is the number of defectives in a random sample of 21 tablets.)

We are told that a shipment will be accepted if at most one tablet doesn't meet the required specifications. That is, the number of defectives must be less than or equal to one i.e

`P\mleft(\mright?\text{shipment }accepted)=P(0)+P(1)_{}`

Substituting we have:

`\begin{gathered} P\mleft(\text{shipment accepted}\mright)=^(21)C_0\cdot\mleft(.01\mright)^0\cdot\mleft(1-.01\mright)^(21-0.)+^(21)C_1\cdot\mleft(.01\mright)^1\cdot\mleft(1-.01\mright)^(21-1). \\ =\text{ }0.80972\text{ + 0.1718} \\ =\text{ 0.98152} \end{gathered}`

Answer:

0.98152