Answer:
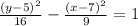
Step-by-step explanation:
The standard form of an hyperbola is:
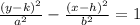
Where (h, k) are the coordinates of the center.
We are given the asyptotes and the foci.
The foci are (7, 0) and (7, 10)
The y value of the center of the parabola is midway from the two foci. Then, the y-coordinate of the center is 5
The coordinated of the center are (7, 5)
Now, we can use that the form of the asymptotes are:

We have:
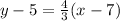
Then:
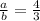
a = 4
b = 3
Now we can write:
![undefined]()