Step-by-step explanation
Part b
So in part (b) we must give the annual growth factor. For any quantity that increases annualy, this factor is the number by which the quantity multiplies itself every year. For a given quantity that increases at a rate of p% its growth factor is given by:

In this case the production of ethanol increases at a rate of 5.5% per year which means that the growth factor is:

Then the growth factor and answer to part (b) is 1.055.
In part (c) we must write an equation that models the increase in the ethanol production. Given an initial qunatity Q and an annual growth factor k the quantity after x years is given by multiplying the initial quantity by the growth factor raised to the x. Then we get:
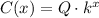
In this case we need a function for the amount of ethanol produced E, x years after 1990. The 0.9 billion gallons produced that year compose our initial quantity and knowing that the annual growth factor is 1.055 we have the desire equation:
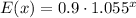
And that's the answer to part (c).
In part (d) we have to compare the actual production of ethanol in 2008 to the one predicted by the equation of part (c). Remember that x represents the years passed after 1990 so for the year 2008 the value of x is given by:

So the number we are looking for is E(18):
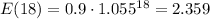
So the production estimated for 2008 is 2.359 billions of gallons. Then the actual production of 9 billion gallons is higher than the one predicted by our equation.