Let's find the lengths and areas of the given figures.
• Question 1
Area of a:
Figure a has the shape of a square.
To find the area, let's find the side length, which is the hypotenuse of triangle b.
Apply Pythagorean Theorem:

Where:
![\begin{gathered} a=\sqrt[]{9}=3m \\ b=\sqrt[]{16}=4m \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/eap3f79ub7vdfr26aasxvehixa6wcnbzw5.png)
Thus, we have:
![\begin{gathered} c^2=a^2+b^2 \\ \\ c^2=3^2+4^2 \\ \\ c^2=9+16 \\ \\ c^2=25 \\ \\ \text{Take the square root of both sides:} \\ \sqrt[]{c^2}=\sqrt[]{25} \\ \\ c=5 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/7abj5dxoh3a53ng71kpny776kuj9jbfvm6.png)
The side length of a is = 5 m
Therefore, to find the area of a, apply the formula for area of a square:
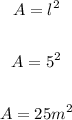
Therefore, the area of a is 25 square meters
• Question 2
Length of b
b is the hypotenuse of the triangle, which is also the side length of figure a.
Therefore, the length of b is 5 m
• Question 3.
Length of C
To find the length of c, apply Pythagorean Theorem:
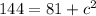
Let's solve for c from the equation:
Subtract 81 from both sides:
![\begin{gathered} 144-81=81-81+c^2 \\ \\ 63=c^2 \\ \\ \text{Take the square root of both sides:} \\ \sqrt[]{63}=\sqrt[]{c^2}^{} \\ \\ 7.94=c \\ \\ c=7.94 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/2x6gylatf8w63kx54nz508fusmjs3mbfgs.png)
Therefore, the length of c is 7.94 m
Question 4:
Area of d.
Figure d is a traingle.
To find the area, apply the formula:
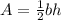
Where:
Base, b = length of c = 7.94
Height, h = √81 = 9 m
Thus, we have:

Therefore, the area of b is 35.73 square meters.
ANSWER:
1. H) 25
2. I) 5
3. A) 7.94
4. B.) 35.73