The question requires that we get the measure of the arc AB
Given: Diameter of circle = 9 feet.
We can then obtain the radius of the circle which is half of the diameter of the circle
Radius = Diameter/2 = 9/2 = 4.5 feet
Length of an arc can be obtained using:
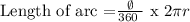
where r = radius
QUESTION 1
Given that the angle subtended by the arc at the center = 45 degrees
We can then substitute the values into the equation
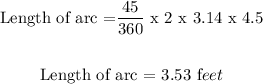
Length of arc = 3.53 feet (To the nearest hundredth )