Before we begin analyzing the question we were asked, we need to compute the probabilities of getting the individual numbers on the spinner out of a total of 12 slots.
Probability of getting a 1:
There is only a single value of 1, therefore the probability of getting a 1 is:
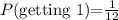
Probability of getting a 2:
There are double values of 2, therefore the probability of getting a 2 is:
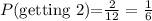
Probability of getting a 3:
There are triple values of 3, therefore the probability of getting a 3 is:
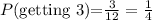
Probability of getting a 4:
There are double values of 4, therefore the probability of getting a 4 is:
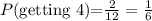
Probability of getting a 5:
There are 4 values of 5, therefore the probability of getting a 5 is:

Now that we know the probabilities of getting the individual numbers we can proceed to solving the questions asked.
A number less than 3 or a 5:
The only numbers less than 3 are: 1 and 2.
Since the spinner is spun just once, it means that, if we are to get a number less than 3, then we get either 1 OR 2.
The probability for getting a 1 OR a 2 is:
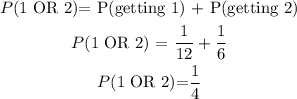
But the question goes on and says in the same trial, what is the possibility of also getting a 5.
This means we can rephrase the question as:
Probability of getting 1 OR 2 OR 5.
Therefore, to fully answer the first question, we say:

The probability of getting a number less than 3 or a 5 is 7/12
Now for the next question;
Spin Even number and then number greater than 3:
To get an even number you can have only: 2 and 4
The question says you spin twice and asks for the probability in which the first number is even. This means the first number is Either 2 OR 4 not both.
We can compute this probability as:

The question also says the second number is a number greater than 3. The only numbers greater than 3 are: 4 and 5.
The second number can be Either 4 OR 5
We can compute this probability as:
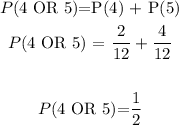
Now that we have both probabilities for the first spin and the second spin, we can therefore calculate for when you get:
Even number AND Number greater than 3
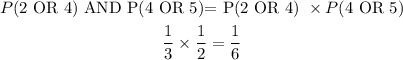
Therefore the probability of getting Even number and number greater than 3 is: 1/6
The final answer is:
#1: 7/12
#2: 1/6