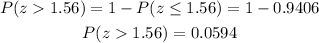Question

Assume that adults have IQ scores that are normally distributed with a mean of 97.1 and a standard deviation of 17.1. Find the probability that a randomly selected adult has an IQ greater than 123.7.

Answer 1

To find the probability you can use the z-score formula, to convert 123.7 into a z-score and then read it on standard normal tables:

`z=(x-\mu)/(\sigma)`

Where:

`\begin{gathered} z\text{ is the z-score} \\ x\text{ is the }value\text{ to evalute 123.7} \\ \mu\text{ is the mean} \\ \sigma\text{ is the standard deviation} \end{gathered}`

By replacing the values you have:

`z=(123.7-97.1)/(17.1)=1.56`

Now, you need to find the probability that an adult has an IQ greater than 123.7, it means.

`P(z>1.56)=1-P(z\leq1.56)`

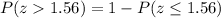
By using the standard normal tables, you will find that P(z<=1.56) is 0.9406:

Replace this value:

`\begin{gathered} P(z>1.56)=1-P(z\leq1.56)=1-0.9406 \\ P(z>1.56)=0.0594 \end{gathered}`