Question

Heather wanted to study how the area of a rectangle changes with the length if its width is fixed. She computed the areas of several rectangles that have the same width and different lengths. She then plotted the results and connected them with a line, as shown below. The graph shows the area (in mm2) versus the length (in mm).Find the range and the domain of the function shown.

Answer 1

Let x and y be the length and area of the rectangle, respectively.

The arrow at the end of the line implies that it keeps growing indefinitely; therefore the range of the function is

`\text{range}=\mleft\lbrace y\in\R|y\ge0\mright\rbrace=y\ge0`

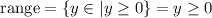
Where we considered that the minimum of the function is at (0,0).

Similarly, the minimum value that x can take is zero because it is a length; then, the domain of the function is

`\text{domain}=\mleft\lbrace x\in\R|x\ge0\mright\rbrace=x\ge0`

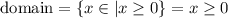
The range is y>=0 and the domain is x>=0