Question

the base of a triangulaf garden is 5 yards longer than the height and the area of the garden is 168 square yards .find a dimension of the triangle

Answer 1

Answer:

The height of the triangle = 16 yards

The base of the triangle = 21 yards

Explanations:

Let the height of the triangle be h

Height = h

The base is 5 yards longer than the height

Base = h + 5

The area = 168 square yards

`\text{The area of a triangle = }(1)/(2)* base* height`
`\begin{gathered} 168\text{ = }(1)/(2)*(h+5)* h \\ h(h+5)\text{ = 2(168)} \\ h^2+5h\text{ = }336 \\ h^2+5h-336\text{ = 0} \\ \end{gathered}`

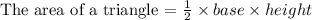
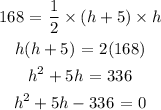
Solve the resulting quadratic equation:

`\begin{gathered} h^2-16h+21h-336=0 \\ h(h-16)+21(h-16)=0 \\ (h-16)(h+21)\text{ = 0} \\ h-16=0 \\ h\text{ = 16} \\ h+21=0 \\ h\text{ = -21} \end{gathered}`

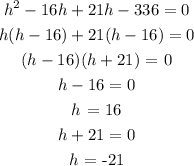
The height of the triangle = 16 yards

The base of the triangle = 16 + 5

The base of the triangle = 21 yards