Question

The lines given by the equations y=4x and y=-0.25x are??

Answer 1

Given:

`The\text{ lines are given by the equations are y=4x and y=-0.25x.}`

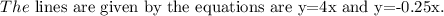
Aim:

We need to find the relationship between the given two lines.

Step-by-step explanation:

Consider the line equation.

`y=mx+b`

where m is the slope.

Compare the line equation y=4x with y=mx+b.

`\text{ Compare the line equation y=4x with }y=m_1x+b.`

`We\text{ get }m_1=4.`

`\text{ Compare the line equation y=-0.25x with }y=m_2x+b.`

`We\text{ get }m_2\text{=-0.25}`

`m_2=-(1)/(4)`
`Substitute\text{ }m_1=4\text{ in the equation.}`

`m_2=-(1)/(m_1).`

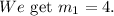
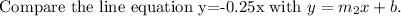
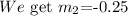
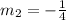
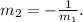
Here the slopes of the given two lines are negative reciprocals of one another.

Recall that perpendicular lines have slopes that are negative reciprocals of one another.

Hence the given two lines are perpendicular to each other.

Final answer:

The lines are given by the equations y=4x and y=-0.25x are perpendicular.