Question

Based on the graph above, estimate (to one decimal place) the averagerate of change from x = 1 to x= 3

Answer 1

Based on the graph above, an estimate of the average rate of change from x = 1 to x= 3 is -0.5.

In Mathematics and Geometry, the average rate of change (ARoC) of a function f(x) on a closed interval [a, b] can be calculated by using this mathematical equation (formula):

Average rate of change (ARoC) =
`(f(b) - f(a))/((b - a))`

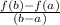
By critically observing the graph of the function f shown below, we can reasonably infer and logically deduce the following:

f(b) = f(3) = 2

f(a) = f(1) = 2.9

Next, we would determine the average rate of change (ARoC) of the function over the interval [1, 3]:

Average rate of change (ARoC) =
`(2 - 2.9)/((3 - 1))`

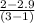
Average rate of change (ARoC) = -0.9/2

Average rate of change (ARoC) = -0.45 ≈ -0.5.