Question

The equations given below are in standard form. Complete both table of values by finding the x and y−intercepts of each equation.

Answer 1

a) x = 6, y = 0

Ordered pair: (6, 0)

x = 0, y = 2

Ordered pair: (0, 2)

b) x = -5, y = 0

Ordered pair: (-5, 0)

x = 0, y = 3

Ordered pair: (0, 3)

Step-by-step explanation:

a) 2x + 6y = 12

`\begin{gathered} x\text{ -intercept is the value of x when y = 0} \\ y-\text{intercept is the value y when x = 0} \end{gathered}`

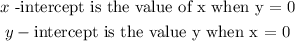
To get x-intercept, we substitute 0 for y:

2x + 6(0) = 12

2x + 0 = 12

2x = 12

x = 12/2

x = 6

x = 6, y = 0

Ordered pair: (6, 0)

To get y-intercept, we substitute 0 for x:

2(0) + 6y = 12

0 + 6y = 12

6y = 12

y = 12/6

y = 2

x = 0, y = 2

Ordered pair: (0, 2)

b) 3x - 5y = -15

x-intercept: y = 0

3x - 5(0) = -15

3x - 0 = -15

3x = -15

x = -15/3

x = -5

x = -5, y = 0

Ordered pair: (-5, 0)

y-intercept: x = 0

3(0) - 5y = -15

0 - 5y = -15

-5y = -15

y = -15/-5

y = 3

x = 0, y = 3

Ordered pair: (0, 3)