1 - exponential growth formula:
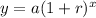
where:
y is the number of Beatles
a is the initial population of beetles
r is the rate (as a decimal)
x is time in moths
Replacing with a = 850, and r = 0.07, we get:
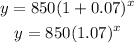
2 - Replacing with x = 11:
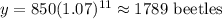
3 - Replacing with y = 100,000:
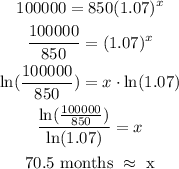
12 months is 1 year, then 70.5 months is equivalent to 70.5/12 = 5.875 years