To determine the type of sequence you have to look at the given amounts.
If this was an arithmetic sequence, the same value will be added to each term in order to determine the next one. If you calculate the difference between two sets of consecutive terms of the sequence, the result must be the same:

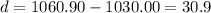
As you can see the differences between the consecutive terms are different, which means that this sequence is not an arithmetic sequence.
To determine a geometric sequence, each term is multiplied by a constant to determine the next term of the sequence. If the given set represents a geometric sequence, then the quotient between two consecutive terms must be equal:
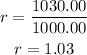
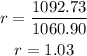
Both quotients are equal which means that this situation represents a geometric sequence.
The common ratio is the result of the quotient between two consecutive terms, so r= 1.03
To calculate the amount in the account for the nth-year, you have to multiply the first term of the sequence by the common ratio raised to n-1:
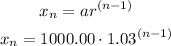
For n=7
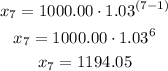
At the end of the seventh year, Maite will have $1,194.05 in the account.