Step-by-step explanation:
The equation for a circle centered at the origin is:
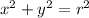
Where r is the radius of the circle.
For this problem it says that it contains point (0, 4). Therefore the radius of the circle is 4 and the equation is:
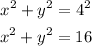
If we replace the given point into the equation and the equality is true, then the point lies on the circle:
![\begin{gathered} (-1)^2+(\sqrt[]{15})^2=16 \\ 1+15=16 \\ 16=16\text{ true} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/y1hky77w3pdhoz94h6bhnb6etvemlf593v.png)
Answer:
Yes, because the equation for the circle is x² + y² = 16 and (-1)² + (√15)² = 16