Given:
sample size (n) = 31
sample mean (bar x) = $44
sample standard deviation = $18
confidence level = 98%
Find: confidence interval
Solution:
To construct a confidence interval, we can use the formula below:
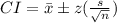
where:
bar x = mean
z = z-value at 98% confidence level
s = sample standard deviation
n = sample size
At 98% confidence level, the z-value is 2.326.
Using the formula above, here are our steps:
a. Divide the sample standard deviation $18 by the square root of sample size 31.
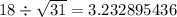
b. Multiply the result to the z-value 2.326.
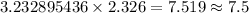
c. Add and subtract 7.5 from the mean of 44.
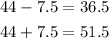
Therefore, the confidence interval at 98% confidence level is from 36.5 to 51.5.