The pulse rate of a person x inches tall is given by the function:
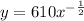
To find the instantaneous rate of change of the pulse rate, we need to find the first derivative of this function and then solve for the x inches tall given.
Follow the next steps:
1. Apply the constant multiple rule of derivatives:
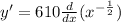
2. Now, to find the derivative apply the power rule of derivatives:
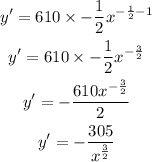
a. A person 37 inches tall will have the following instantaneous rate of change of the pulse rate:
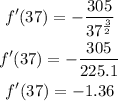
b. A person 63 inches tall will have the following instantaneous rate of change of the pulse rate:
