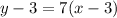Answer:
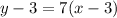
Explanation:
Pre-Solving
We are given that a line has a slope (m) of 7, and it contains the point A.
Point A has the coordinates of (3,3).
We want to write the equation of this line.
The equation of the line can be written in three different ways:
- Slope-intercept form, which is y=mx+b, where m is the slope and b is the y intercept.
- Standard form, which is ax+by=c, where a, b, and c are free integer coefficients. a and b cannot be to 0, and a is usually non-negative.
- Point-slope form, which is
 , where m is the slope and
, where m is the slope and
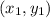 is a point.
is a point.
Since the question does not specify that the equation had to be written in a specific way, any of these ways will work.
However, for the sake of this problem, let's write the equation in point-slope form, since it is the easiest.
Solving
Since we are already given the slope of this line, we can immediately plug it into
 .
.
Replace m with 7.
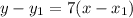
Let's label the values of the point A to avoid confusion / mistakes while calculating.
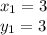
Substitute the values above into our equation.