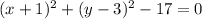Using the general equation for a circle, we have:
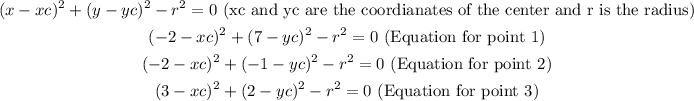
Then, we are going to subtract the first equation from the second and the first from the third to get a system of equations. Doing so, we have:
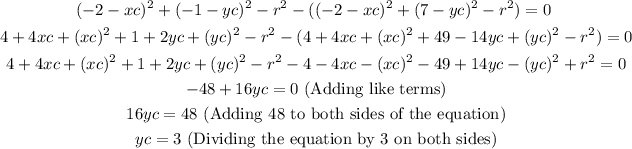

We found that the coordinates of the center are (-1,3), Replacing them in the first equation to find the radius, we have:

Knowing that the center is at (-1,3) and the radius is 4.12, the general equation would be: