Answer:
4 units to the right and 4 units down.
Step-by-step explanation:
Given the pre-image point A(4,1)
The translation from A to A' is shown below:
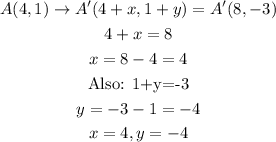
• Since ,4 is added to the unit on the ,x-axis, we say it is translated 4 units to the right.
,
• Since ,4 is subtracted to the unit on the y-axis,, we say ,it is translated 4 units down.
We conclude that point A is translated 4 units to the right and 4 units down.