In this problem, we are trying to interpret a set of points to determine the domain and range, and if it is a function.
Domain: The domain of a function is all the possible x-values. In this case, the x-coordinates.
Range: The range of a function is all the possible y-values, or x-coordinates.
We are given the following points:
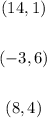
To find the domain, we just need the x-values:
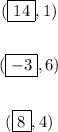
Our domain is: -3, 8, 14 (in numeric order).
We can follow the same procedure for the range:
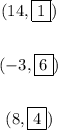
Our range is: 1, 4, 6, in numeric order.
Finally, we need to know if the set of points is a function.
We know if a set of points is a function when no x-values are repeated with different y-values. We see form our points that the domain has no repeating x-values.
Therefore, yes, this set of points shows a function.