Question

Brad's average for five quizzes is 86If he wants to have an average of 88for six quizzes, what is the lowestscore he can receive on his sixth quiz?

Answer 1

Let the sum of Brad's quizzes score be x.

Since

`\text{average = }\frac{s\text{um of quiz scores }}{n\text{umber of quizzes}}`

It means that

`\begin{gathered} 86=(x)/(5) \\ \text{for five quizzes } \end{gathered}`

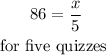
Let the score of the sixth quiz be y, so this means that

`88=(x+y)/(6)`

From the first equation, we have x as

`\begin{gathered} 86=(x)/(5) \\ \text{cross multiply, we have } \\ x=86*5 \\ x=430 \end{gathered}`

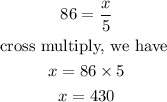
Now we will substitute x for 430 into the second equation, we have

`\begin{gathered} 88=(x+y)/(6) \\ 88=(430+y)/(6) \\ \text{cross multiply, we have } \\ 430+y=88*6 \\ 430+y=528 \\ \text{collecting like terms, y becomes } \\ y=528-430 \\ y=98 \end{gathered}`

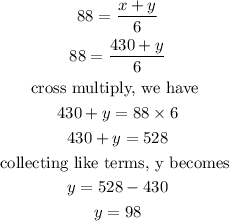
Hence the answer is 98