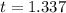We are given the size of the sample:
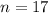
To find the critical t value, we will need to find "df"--> the degrees of freedom. This is defined as the size of the sample minus 1:

Now, we need to look in a t-table the point where df=16 and the confidence level is 80%. We can see this in the following image:
The t critical value is:
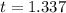
Answer: