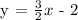Given the following coordinates of the two points that pass through the line.
Point 1 : 0, - 2
Point 2 : 2, 1
Let's determine the equation of the line:
Step 1: Let's determine the slope (m).

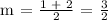
Step 2: Let's determine the y - intercept (b). Using the slope-intercept form: y = mx + b, plug in m = 3/2 and x,y = 0, -2.

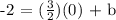
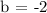
Step 3: Let's complete the equation. Plug in m = 3/2 and b = -2 in y = mx + b.

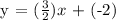
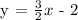
Therefore, the equation of the line is: