Question

If you invest $10,829.00 into an account earning an annual nominal interest rate of 7.042%, how much will you have in your account after 11 years if the interest is compoundedquarterly? If the interest is compounded continuously?If interest is compounded quarterly: FV= ___If interest is compounded continuously: FV= ___What is the Effective Annual Yield in percent when the annual nominal interest rate is 7.042% compounded quarterly?EAY = ____%(Note: All answers for FV = should include a dollar sign and be accurate to two decimal places)

Answer 1

Given:

If you invest $10,829.00 into an account earning an annual nominal interest rate of 7.042%

`\begin{gathered} P=10,829 \\ R=7.042 \\ n=4\text{ year} \\ t=11 \end{gathered}`

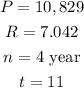
Required:

To fid the amount after 11 years if the interest is compounded quarterly, and If the interest is compounded continuously.

Step-by-step explanation:

Now,

`\begin{gathered} R=7.042\% \\ \\ =(7.042)/(100) \\ \\ =0.07042 \end{gathered}`

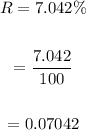
Therefore, The compound interest after 11 year is,

`A=P(1+(r)/(n))^(nt)`
`\begin{gathered} =10,829(1+(0.07042)/(4))^(4(11)) \\ \\ =23,338.59 \end{gathered}`

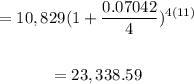
So $23,338.59 will have in account after 11 years if the interest is compounded quarterly.

Now,

`\begin{gathered} A=Pe^(rt) \\ \\ =10,829* e^(0.07042(11)) \\ \\ =10,829*(2.71828)^(0.07042(11)) \\ \\ =23,496.41 \end{gathered}`

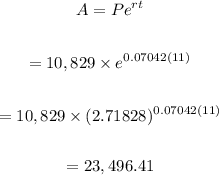
So, $29,496.41 will have in account after 11 years If the interest is compounded continuously.

Final Answer:

$23,338.59 will have in account after 11 years if the interest is compounded quarterly.

$29,496.41 will have in account after 11 years If the interest is compounded continuously.