Question

I need help finding the correlation coefficient I got everything else

Answer 1

From the given table, the equation of the line of best fit is:

`y=13.98x+478.73`

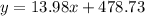
where x denotes the years and y the number of students.

Now, the mean of the y-values is

`\mu_y=(5556)/(10)=555.6`

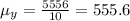
The variance of y-values is given by

`\text{SSy}=\Sigma(y-\mu_y)^2`

By expanding this sum, we have

`\text{SSy}=(492-555.6)^2+(507-555.6)^2+(520-555.6)^2+\ldots+(618-555.6)^2`

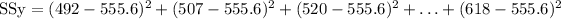
which gives

`\text{SSy}=16118.4`

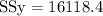
By doind the same process for the varible x, we have that the correlation coefficient R is given by:

`R=0.9999`

since this result is near to 1, there is a strong positive relationship between the year and the number of students