Question

Hi, can you help me to solve this exercise please!

Answer 1

The triangle in the picutre is a right triangle, because it has a right angle.

The side opposite the right angle is called hypotenuse and the other two are the legs.

We also know on of the other angles, 30°, and its opposite side, 5.

Since we know one angle and its opposite side, we can find the hypotenuse using the sine of the angle, because the sign of an angle in a right triangle is equal to the opposite leg divided by the hypotenuse.

So, the equation we can use is:

`\sin 30\degree=(5)/(x)`

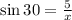
To actually find the value of x, we first solve for it:

`\begin{gathered} \sin 30\degree=(5)/(x) \\ x=(5)/(\sin 30\degree) \end{gathered}`

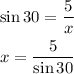
And now, we input the value of sin 30°, which is known:

`\sin 30\degree=(1)/(2)`

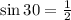
So:

`x=(5)/(\sin30\degree)=(5)/((1)/(2))=5\cdot2=10`

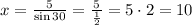
The value of x is 10.