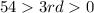Question

you're getting two of the side lengths of triangles 27 mm and 27 what is the solution range for the 3rd side length ?

Answer 1

In a triangle, one of the sides must be greater than the difference of the other 2 sides and less than the sum of these.

Which means that the 3rd side must be greater than 27-27 and less than 27+27.

`\begin{gathered} 27-27=0 \\ 27+27=54 \end{gathered}`

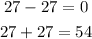
The 3rd side length must be greater than 0 but less than 54. This is:

`54>3rd>0`