Answer:
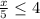
or also;
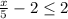
Step-by-step explanation:
Given the inequality;
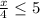
solving the inequality, we have;
multiply both sides by 4;
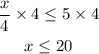
The inequality has a solution of x=p.
So,
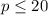
We want to write an inequality that also falls within the range of this solution.
Examples of equations that also have their solution within this range are;
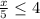
also;
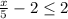
Any of the two inequalities above also has a solution of x=p.