Question

Anna purchased how many adult tickets Then how much did she spend on child tickets

Answer 1

Given that the children's tickets cost $9 each and the adult tickets cost $19 each. The total number of tickets purchased is 72, and the total amount spent on all the tickets is $808

To Determine: The number of tickets purchased for the children and adult tickets

Solution:

Let the number of tickets purchased for the children be x and the number of tickets purchased for the adult be y

Given that the total number of tickets purchased is 72. Then

`x+y=72====\text{equation 1}`

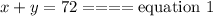
If the children's tickets cost $9 each. Then the total amount spent to purchase children's tickets would be

`A_{\text{children's tickets}}=x*9=9x`

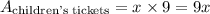
If the adult's tickets cost $9 each. Then the total amount spent to purchase adult's tickets would be

`A_{\text{adult's tickets}}=y*19=19y`

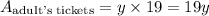
The total amount of money spent to get children and adult tickets is $808. Then,

`9x+19y=808====\text{equation 2}`

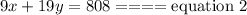
Combining equation 1 and equation 2

`\begin{gathered} x+y=72====\text{equation 1} \\ 9x+19y=808====\text{equation 2} \\ \text{From equation 1,} \\ x=72-y \end{gathered}`

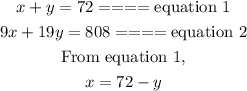
Substitute x in equation 2

`\begin{gathered} 9(72-y)+19y=808 \\ 648-9y+19y=808 \\ -9y+19y=808-648 \\ 10y=160 \\ \text{divide through by 10} \\ (10y)/(10)=(160)/(10) \\ y=16 \end{gathered}`

Substitute for y in x

`\begin{gathered} x=72-y \\ x=72-16 \\ x=56 \end{gathered}`

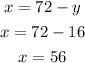
The total amount spent to purchase children's and adult tickets would be

`\begin{gathered} 9x=9*56=504 \\ 19y=19*16=304 \end{gathered}`

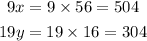
Hence:

Anna purchased 16 adults tickets,

Anna purchased 56 children's tickets

The cost of children's tickets is $504

The cost of adult tickets is $304