The degree of the polynomial is 5 while the number of terms in the polynomial is 3.
To solve this problem
Let's identify the degree and number of terms of the polynomial:

Degree:
The degree of a polynomial is the highest power of the variable in the polynomial. In this case, the highest power of the variable is 5 (in the term
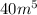 ).
).
Therefore, the degree of the polynomial is 5.
Number of terms:
A term is a single part of a polynomial that is separated by addition or subtraction signs. In this case, there are three terms:
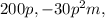 and
and
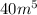 .
.
Therefore, the number of terms in the polynomial is 3.