Question

(3x^2+25x+8)/(x^2+7x-8)find the x intercept (s) or explain why there is none

Answer 1

(-1/3, 0)

Step-by-step explanation:

To find the x-intercepts, we need to make the function equals to zero and solve for x, so:

`(3x^2+25x+8)/(x^2+7x-8)=0`

Now, we need to factorize the numerator and the denominator as follows:

`(3x^2+25x+8)/(x^2+7x-8)=((3x+1)(x+8))/((x-1)(x+8))=0`

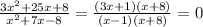
So, we can simplify the expression as:

`(3x+1)/(x-1)=0`

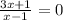
Finally, a division is equal to zero only if the numerator is equal to zero. So:

`\begin{gathered} 3x+1=0 \\ 3x+1-1=0-1 \\ 3x=-1 \\ (3x)/(3)=-(1)/(3) \\ x=-(1)/(3) \end{gathered}`

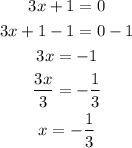
Therefore, the x-intercept of the function is the point (-1/3, 0)