Question

Functions 1 and 2 are shown below. Which statement about the rate of change iscorrect?Function 1Function 2y = kx + 1(1.4)y - 3x + 1321(0,1)13-2123-1-2-34Function 1 has a greater rate of change.Function 2 has a greater rate of change.Both functions have the same rate of change.There is not enough information to determine which function has the greater rate ofchange

Answer 1

Function 1 has a greater rate of change

Step-by-step explanation

We have two functions in the question.

`y\text{ = 3x + 1}`

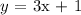
and

`y\text{ = }(1)/(3)x\text{ + 1}`

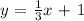
The rate of change of a function is defined as the slope or gradient of the function.

Linear functions (such as these) have a general form:

y = mx + c

where m = slope/gradient

c = y intercept

By comparing, we see that:

For function 1, m = 3

For function 2, m = 1/3

Therefore, the gradient (rate of change) of function 1 is greater than that of function 2.