Question

#10. If the nominal rate on a CD is 3.8%, what is the effective rate? Assume monthlycompounding, and round your answer to the nearest hundredth of a percent.

Answer 1

The effective rate is of 3.87%.

Explanation:

The effective rate, as a decimal, is given by the following formula:

`i=(1+(r)/(m))^m-1`

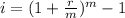
In which r is the nominal rate, as a decimal, and m is the number of compoundings a year.

In this question:

Nominal rate of 3.8%, so r = 0.038

Monthly compounding, so 12 times a year, which means that m = 12. Then

`i=(1+(r)/(m))^m-1=(1+(0.038)/(12))^(12)-1=0.0387`

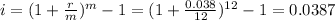
0.0387*100 = 3.87%

The effective rate is of 3.87%.