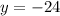Question

What is an equation of the line tangent to f(x) = 3(2x² - 4x)^3 at point (1, - 24)?

Answer 1

Given:

The equation of the curve is given as,

`f(x)\text{ = 3\lparen2x}^2-4x)^3`

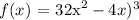
The required line is the tangent to the given curve at the point (1, -24).

Required:

Equation of the line tangent to the given curve.

Step-by-step explanation:

Differentiating the given function with respect to x,

`\begin{gathered} f^(\prime)(x)\text{ = 3}(d)/(dx)\left(2x^2-4x\right)^3 \\ f^(\prime)(x)=3*3*\left(2x^2-4x\right)^2*(d)/(dx)\left(2x^2-4x\right) \\ f^(\prime)(x)=9\left(2x^2-4x\right)^2(4x-4) \end{gathered}`

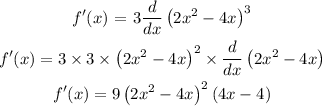
Slope of the tangent at (1,-24) is calculated as,

`\begin{gathered} f^(\prime)(x)\text{ at \lparen1,-24\rparen = 9}*[2\left(1\right)^2-4(1)]^2*[4(1)-4] \\ f^(\prime)(x)\text{ at \lparen1,-24\rparen = 9}*[2-4]*[4-4] \\ f^(\prime)(x)\text{ at \lparen1,-24\rparen = 9}*-2*0\text{ } \\ f^(\prime)(x)\text{ at \lparen1,-24\rparen = 0} \\ \end{gathered}`

Equation of the required line is given as,

`\begin{gathered} (y-(-24))=0(x-1) \\ y+24\text{ = 0} \\ y\text{ = -24} \end{gathered}`

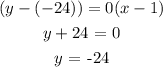
Answer:

Thus the equation of the required line is

`y=-24`