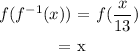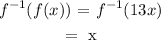Question

Verify the equation is correct and fill in the blanks for B

Answer 1

Given that the function

`f(x)\text{ =13x}`

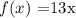
is a one-to-one function,

A) Equation for f⁻¹(x), the inverse function.

From the function f(x), interchange x and f(x).

thus,

`\begin{gathered} f(x)\text{ = 13x} \\ in\text{terchanging x and f(x), we have} \\ x\text{ = 13f(x)} \\ \text{divide both sides by 13} \\ f(x)\text{ = }(x)/(13) \end{gathered}`

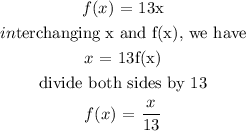
thus, equation for f⁻¹(x), the inverse function is

`f(x)\text{ = }(x)/(13)`

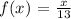
B) To show that f(f⁻¹(x)) = x and f⁻¹(f(x)) = x

Starting with f(f⁻¹(x)):

Evaluating f(f⁻¹(x)) involves substituting the f⁻¹(x) function into the f(x) function.

In this case, let f⁻¹(x) be z(x).

Thus, we have f(f⁻¹(x)) to be f(z).

`\begin{gathered} z(x)=f^(-1)(x)\text{ = }(x)/(13) \\ f(x)\text{ = }13x \\ \text{thus, substituting the z(x) function into the f(x) function, we have} \\ f(z(x))\text{ = }13((x)/(13)) \\ \Rightarrow x \\ \end{gathered}`

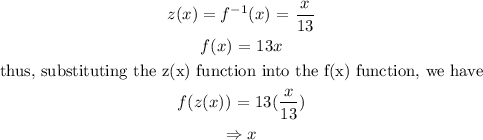
For f⁻¹(f(x)):

Similarly, evaluating f⁻¹(f(x)) involves substituting the f(x) function into the f⁻¹(x) function.

Thus, we have

`\begin{gathered} f(x)\text{ = 13x} \\ f^(-1)(x)\text{ = }(x)/(13) \\ \text{thus, substituting f(x) into }f^(-1)(x)\text{ gives} \\ f^(-1)(f(x))\text{ = }(1)/(13)(13x) \\ \Rightarrow x \\ \end{gathered}`

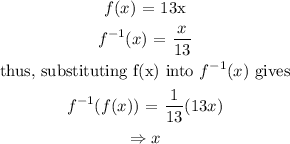
Hence,

`\begin{gathered} f(f^(-1)(x))\text{ = }f((x)/(13)) \\ \text{ = x} \end{gathered}`
`\begin{gathered} f^(-1)(f(x))\text{ = }f^(-1)(13x) \\ =\text{ x} \end{gathered}`