In order to determine the amount of the trace element, proceed as follow:
First calculate the volume of the cylindric container by using the following formula:

where:
r: radius of the base of the cylinder = 8.6 cm
h: height = 11 cm
Replace the previous values into the formula for V and simplify:

Now, consider that there are 0.027 grams of the trace element in one cubic centimeter. Then, for the previous volume you have:
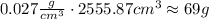
Hence, there area approximately 69 grams of the trace element into the cylindric container.